Question

Use the fact that the world population was 2560 million in 1950 and 3040 million in 1960 to model the population of the world in the second half of the 20th century. (Assume that the growth rate is proportional to the population size.) What is the relative growth rate? Use the model to estimate the world population in 1991 and to predict the population in the year 2020.

Answer 1

The populaton in year 1991 will be of 5178.9 million of people

and the population in year 2020 will be of 8524.60 million of people

Explanation:

We measure the time t in years and let t=0 in the year 1950.

We measure the population P(t) in millions of people, then P(0)=2560 and P(10) = 3040.

Since we are assuming that dp/dt=kP, this theorem gives the following.

`P(t) = P(0)e^(kt)= 2560e^(kt)`

`P(10) = 2560 e^(10k) = 3040`

`k=(1)/(10) ln (3040/2560) = 0.0171`

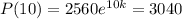
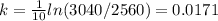
The relative growth rate is about 1.7% per year and the model is

`P(t) = 2560e^(0.017185t)`

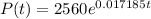
Year 1991 is equal in our formula to 41 and year 2020 is equal to 70. Replacing,

`P(41) = 2560e^(0.017185(41))=5178.89`

`P(70) = 2560e^(0.017185(70))=8524.60`

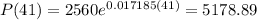
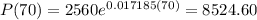
Therefore the populaton in year 1991 will be of 5178.9 million of people and the population in year 2020 will be of 8524.60 million of people