Question

Adecco Workplace Insights Survey sampled men and women workers and asked if they expected to get a raise or promotion this year (USA Today, February 16, 2012). Suppose the survey sampled 200 men and 200 women. If 104 of the men replied yes and 74 of the women replied yes, are the results statistically significant in that you can conclude a greater proportion of men are expecting to get a raise or a promotion this year?

Answer 1

Yes

Explanation:

Let p1 represent proportion of women and p2 proportion of men. The null and alternative hypothesis will be as follows

Null hypothesis

`H_o`=p2-p1 ≤0

Alternative hypothesis

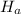`H_a`=p2-p1>0

Sample proportion of women, p1=74/200=0.37

Sample proportion of men is p2=104/200=0.52

Level of significance is 0.01

Pooled proportion=
`\frac {104+74}{200+200}=0.445`

Test statistic

`z=\frac {(0.52-0.37)-0}{0.445(1-0.445)*√((1/200+1/200))}=6.9124`

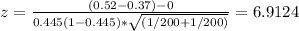
p-value=P(Z≥z)=P(Z≥6.9124)=P(Z≤-6.9124)=0

Since the value of p is less than 0.01, we reject null hypothesis. There’s sufficient evidence that a greater proportion of men is expecting to get a raise