Answer:
About 89 bicycles.
Explanation:
Let C(x) is average cost (in hundreds of dollars) per bicycle and x be the number bicycles built (in hundred).

We need to find the number of bicycles for which the average cost per bicycle is minimum.
The vertex form of a parabola is
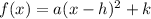 .... (1)
.... (1)
where, a is constant and (h,k) is vertex.

Taking out 0.9 from the parenthesis.
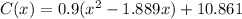
If an expression is
 , then we add
, then we add
 in the expression to make it perfect square.
in the expression to make it perfect square.
Here, b=-1.889,
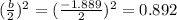
Add an d subtract 0.892 in the parenthesis.
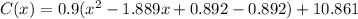
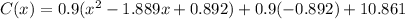
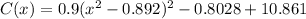
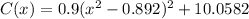 ... (2)
... (2)
From (1) and (2) we get
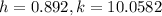
Aki should built 0.892 hundred bicycle to minimize the average cost per bicycle.
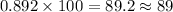
Therefore, the Aki should built 89 bicycle to minimize the average cost per bicycle.