Question

A glider has a mass m = 0.4 kg and is resting on a track that has a small but finite coefficient of kinetic friction. It is attached to a spring with force constant k = 20 N/m. The relaxed position of the spring corresponds to x= 0. The glider is pulled out to x = 0.2 m and then released. If its speed is 1.0 m/s when it first reaches x = 0.1 m, determine the work done by friction during that segment of the motion.

Answer 1

-0.1 Joules

Step-by-step explanation:

Here, the kinetic energy of the spring and glider is conserved.

So, we get

KE of spring + Work done by friction = Change in momentum of glider

`(1)/(2)k(x_f^2-x_i^2)+W_f=(1)/(2)m(v^2-u^2)\\\Rightarrow W_f=(1)/(2)m(v^2-u^2)-(1)/(2)k(x_f^2-x_i^2)\\\Rightarrow W_f=(1)/(2)0.4(1^2-0^2)-(1)/(2)20(0.2^2-0.1^2)\\\Rightarrow W_f=-0.1\ Joules`

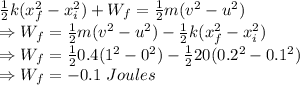
The work done by friction during that segment of motion is -0.1 Joules