Answer:
-2.63 Joules
2.63 Joules
Step-by-step explanation:
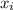 = Initial compression = 5.89 cm
= Initial compression = 5.89 cm
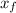 = Final compression = -15.4 cm
= Final compression = -15.4 cm
k = Spring constant = 260 Nm
Work done by a spring is given by

Work done by the spring is -2.63 Joules.
Change in kinetic energy is given by
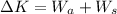
Here, it is assumed that change in kinetic energy is zero as velocity and amlitude are not mentioned.
So,
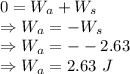
The work done by the applied force is 2.63 Joules.