Question

What is the value of x?

Enter your answer in the box.

x =

Answer 1

I think the value of x may be 1. I’m not entirely sure, though.

Answer 2

`x=19`

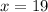
Explanation:

We have been given a diagram of two intersecting lines. We are asked to find the value of x.

We know that measure of two vertical angles is always equal.

We can see that angle
`3x-3` and
`6(x-10)` are vertical angles, so we will equate measure of both angles and solve for x as:

`6(x-10)=3x-3`

`6\cdot x-6\cdot 10=3x-3`

`6x-60=3x-3`

`6x-60+60=3x-3+60`

`6x=3x+57`

`6x-3x=3x-3x+57`

`3x=57`

`(3x)/(3)=(57)/(3)`

`x=19`

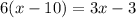
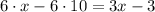
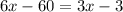
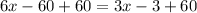
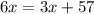
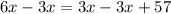
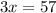
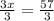
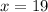
Therefore, the value of x is 19.