Question

Use the equation y=s x 4 −16 s 3 y=sx4−16s3 to answer the question. How can you rewrite the given formula to correctly isolate x x ? Having trouble understanding how to isolate it

Answer 1

`x=\sqrt[4]{(y+16s^3)/(s)}`

Explanation:

It appears you intend your equation to be ...

`y=sx^4-16s^3`

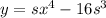
In any "solve for" situation, it helps to consider what is being done to the variable of interest. (The order of operations is useful here.) In this case, ...

• x is raised to the 4th power
• the result is multiplied by s
• that product has 16s³ subtracted from it

You can isolate the variable by "undoing" these operations in reverse order.

The subtraction of 16s³ can be undone by adding 16s³. Anything we do must be done to both sides of the equation, so this gives ...

`y+16s^3=sx^4 \qquad\text{add $16s^3$}\\\\(y+16s^3)/(s)=x^4 \qquad\text{divide by the coefficient of the x-term}\\\\\sqrt[4]{(y+16s^3)/(s)}=x \qquad\text{take the fourth root to undo the power}`