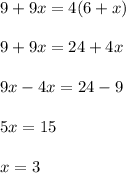Question

How many liters of a 90% acid solution must be added to 6 liters of a 15% acid solution to obtain a 40% acid solution?

Answer 1

3 liters

Explanation:

Let x liters of 90% acid solution is added to 6 liters of a 15% acid solution to obtain a 40% acid solution.

There are
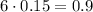`6\cdot 0.15=0.9` liter of acid in 6 liters of 15% acid solution

There are
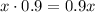`x\cdot 0.9=0.9x` liter of acid in x liters of 90% acid solution

There are
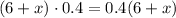`(6+x)\cdot 0.4=0.4(6+x)` liter of acid in 6 + x liters of 40% acid solution

So,

`0.9+0.9x=0.4(6+x)`

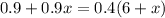
Solve this equation

`9+9x=4(6+x)\\ \\ 9+9x=24+4x\\ \\9x-4x=24-9\\ \\5x=15\\ \\x=3`