Question

A suspension bridge has two main towers of equal height. A visitor on a tour ship

approaching the bridge estimates that the angle of elevation to one of the towers
is 15°. After sailing 497 ft closer he estimates the angle of elevation to the same
tower to be 42° Approximate the height of the tower.

Answer 1

The Height of the tower is 188.67 ft

Explanation:

Given as :

The angle of elevation to tower = 15°

The distance travel closer to tower the elevation changes to 42° = 497 ft

Now, Let the of height of tower = h ft

The distance between 42° and foot of tower = x ft

So, The distance between 15° and foot of tower = ( x + 497 ) ft

So, From figure :

In Δ ABC

Tan 42° =
`(perpendicular)/(base)`

Or , Tan 42° =
`(AB)/(BC)`

Or, 0.900 =
`(h)/(x)`

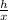
∴ h = 0.900 x

Again :

In Δ ABD

Tan 15° =
`(perpendicular)/(base)`

Or , Tan 15° =
`(AB)/(BD)`

Or, 0.267 =
`(h)/(( x + 497 ))`

Or, h = ( x + 497 ) × 0.267

So, from above two eq :

0.900 x = ( x + 497 ) × 0.267

Or, 0.900 x - 0.267 x = 497 × 0.267

So, 0.633 x = 132.699

∴ x =
`(132.699)/(0.633)`

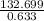
Or, x = 209.63 ft

So, The height of tower = h = 0.900 × 209.63

Or, h = 188.67 ft

Hence The Height of the tower is 188.67 ft Answer