Answer:

Explanation:
The two triangles are similar. So that means it's corresponding sides are proportional.
So this means we can find x using the equation:
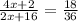
Simplify:
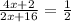
Cross multiply:
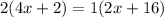
Distribute/multiply:
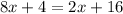
Subtract
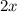 on both sides:
on both sides:
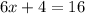
Subtract
 on both sides:
on both sides:
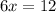
Divide both sides by 6:
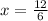
Simplify:
