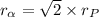Answer:
(a)
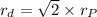
(b)
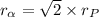
Step-by-step explanation:
charge on proton, q = e
mass pf proton, m = mp
charge on deuteron, q' = e
mass pf deuteron, m' = 2mp
charge on alpha particle, q'' = 2e
mass pf alpha particle, m'' = 4mp
Potential difference = V
Magnetic field = B
The kinetic energy is given by eV.
So, 1/2 mv^2 = e V
where, v be the velocity of the particle.
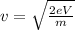
The formula for the radius of circular path is given by

By substituting the value of v
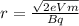
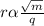
The radius of path of proton is given by
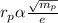 .... (1)
.... (1)
(a) radius of deuteron
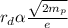
By comparing equation (1), we get
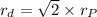
(b) radius of alpha particle
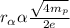
By comparing equation (1), we get