Answer:
(a) 43.6°
(b) 66.5°
Step-by-step explanation:
Refractive index for plastic np = 1.45
refractive index of air na = 1
(a)
Let the maximum value of angle of incidence in air is i and the angle of refraction is 90° for total internal reflection.
By using Snell's law
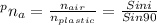
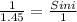
Sin i = 0.6897
i = 43.6°
(b)
Let the maximum value of angle of incidence in water is i and the angle of refraction is 90° for total internal reflection.
By using Snell's law
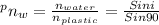
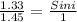
Sin i = 0.917
i = 66.5°