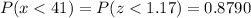Answer:
The probability that there will be at most 41 correct answers is 0.8790
Explanation:
We can aproximate the probability by using a binomial distribution where:
p: A question on a test is answered correctly
n: number of responses
So, the mean of the distribution is given by:
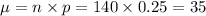
and the standar deviation is given by:

The normalized variable for 41 correct answers is:
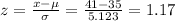
Hence, the probability that there will be at most 41 correct answers is: