Question

A sprinkler distributes water in a circular pattern, supplying water to a depth of e^-r feet per hour at a distance of r feet from the sprinkler.

A) what is the total amount of water supplied per hour inside of a circle of radius 11?
B) what is the total amount of water that goes throughout the sprinkler per hour?

Answer 1

A) 12,5651 feet/hour

B) 12,5663 feet/hour

Explanation:

A) Being

f
`f(x)=e^(-r)`

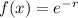
the amount of water distributed at a distance r. But, the total amount of water distributed inside a circle of radius r , is the sum of all the water distributed from 0 until r. That is

`W(R=11)=\int\limits^R_(-R)\int\limits^R_(-R) {f(x,y)} \, dxdy = \int\limits^(2\pi) _(0)\int\limits^R_(0) {f(x,y)} \, r drd\alpha = \int\limits^(2\pi) _(0)\int\limits^R_(0) e^(-r) \, r drd\alpha = 2\pi [2-(R+1)e^(-R) ] = 2\pi [2-(11+1)e^(-11) ] = 12,5651`

B) the total ammount of water that goes out of the sprinkler will be distributed to different distances according to f(r) , therefore it will be the sum of all the ammount of water at all the distances.

`W(R=\infty)= \lim_(R \to \infty) 2\pi [2-(R+1)e^(-R) ] =2\pi [2- \lim_(R \to \infty)(R+1)e^(-R) ] = 2\pi [2- \lim_(R \to \infty)(R+1)/e^(R) ]  = 2\pi [2- \lim_(R \to \infty) 1/e^(R) ]  = 2\pi [2- 0] = 4\pi = 12,5663`