Question

Confidence Interval Given. Assume I created a 95% confidence interval for the mean hours studied for a test based on a random sample of 64 students. The lower bound of this interval was 4 and the upper bound was 14. Assume that when I created this interval I knew the population standard deviation. Using this information, (a) Calculate the width of the interval. (b) Calculate the margin of error for the interval. (c) Calculate the center of the interval. (d) What is the sample mean? (e) What is the z ∗ (or zα/2) used? (f) Calculate the population standard deviation. [

Answer 1

Explanation:

Given that confidence Interval Given. Assume I created a 95% confidence interval for the mean hours studied for a test based on a random sample of 64 students.

Lower bound =4

Upper bound =14

Hence mid value = Mean =
`(4+14)/(2) =9`

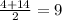
a) Width of interval =upper bound -Lower bound =
`14-4=10`

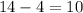
b) Margin of error =
`14-mid value = 5`

c) Center of interval =average =9

d) Sample mean = center of interval =9

e) Z value for 95% would be
`1.96`

f) Population std deviation = Margin of error/Z value

=
`(5)/(1.96) \\=2.5510`