Question

An ideal 4.7uF capacitor is charged to 5 volts then removed from the charging circuit and checked for voltage with a real DMM. At first the DMM reads 5 volts, but then the voltage starts dropping. After half a minute the voltage is down to 2.5 volts and still dropping. (a) why? (hint: it has something to do with the DMM) (b) in light of the hint above, what quantitative piece of information can you deduce concerning the DMM?

Answer 1

so that DMM has an internal resistance of 9.21 M ohm

Step-by-step explanation:

given data

capacitor = 4.7 uF

charged = 5 volts

half a minute voltage down = 2.5 volts

solution

we know that capacitor has discharge path

so DMM act as discharge path since the capacitor is an ideal

and

V initial is 5 v and V final is 0 v

so that

Vc = V final + ( Vinitial - V final )
`e^{(-t)/(\tau) }`

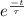
Vc = 0+ 5
`e^{(-t)/(\tau) }`

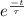
τ = Reg C , (4.7 u) Reg

Vc = 2.5 V at 30 seconds

so that

2.5 = 5
`e^{(-30)/(\tau) }`

solve we get τ = 43.28

so that

Reg ( 4.7 u ) = 43.28

Reg = 9.208 M ohm

so that DMM has an internal resistance of 9.21 M ohm