Question

In analyzing hits by certain bombs in a​ war, an area was partitioned into 556 ​regions, each with an area of 0.45 km2. A total of 545 bombs hit the combined area of 556 regions. Assume that we want to find the probability that a randomly selected region had exactly two hits. In applying the Poisson probability distribution​ formula, ​P(x)=μ^x * e^-μ/ x!​, identify the values of μ​, ​x, and e. ​Also, briefly describe what each of those symbols represents.

Answer 1

x is the number of sucesses

e = 2.71828 is the Euler number

`\mu` is the mean in the given interval.

There is a 18.03% probability that a randomly selected region had exactly two hits.

Explanation:

In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

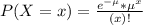
In which

x is the number of sucesses

e = 2.71828 is the Euler number

`\mu` is the mean in the given interval.

In this problem we have that:

A total of 545 bombs hit the combined area of 556 regions. So the mean hits per region is:

`P = (545)/(556) = 0.9802`

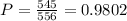
Assume that we want to find the probability that a randomly selected region had exactly two hits.

This is P(X = 2).

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

`P(X = 2) = (e^(-0.9802)*(0.9802)^(2))/((2)!) = 0.1803`

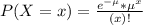
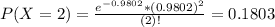
There is a 18.03% probability that a randomly selected region had exactly two hits.