Answer:
The rate at which the population decrease is 59.8 %
Explanation:
Given as :
The population of city = 30,31,000
Now The population of city decrease to 22,46,000
The time period in which population decrease is 50 years
Let the percentage rate of decrease = R%
So,
Final population = initial population ×
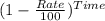
Or, 22,46,000 = 30,31,000 ×
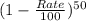
Or,
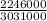 =
=
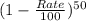
Or,
 =
=
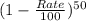
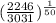 =
=

So , 0.99402 =

Or,
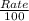 = 1 - 0.99402
= 1 - 0.99402
So, Rate =
 × 100
× 100
Or, Rate = 0.598 = 59.8 %
Hence The rate at which the population decrease is 59.8 % Answer