Answer:
dh/dt = 0.107m/min
dr/dt = 0.1435m/min
Step-by-step explanation:
The volume of a cone is given by:
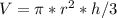
The relationship between height and radius is:
h = 3/8 * (2*r) = 3/4 * r
Replacing this value into the volume formula:
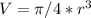
If we derive this equation:
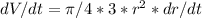 Solving for dr/dt and knowing that dV/dt=15m^3/min
Solving for dr/dt and knowing that dV/dt=15m^3/min
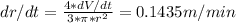
With the relation between height and radius:
dh/dt=3/4*dr/dt = 0.107m/min