Question

Increasing the surface temperature of a ball bearing for a short time without significantly raising the temperature inside the ball bearing produces a hard outer surface desirable in ball bearings. For a steel (r = 7800 kg/m3; Cp = 500 J/kg*K; k = 50 W/m*K) ball bearing, assume that any location within the bearing where the temperature is greater than 1000 K will be hardened. The ball bearing, initially at 300 K, will be placed in a very hot (1300 K) fluid with an extremely high convection coefficient (5,000 W/m2*K). Determine the time required for the outer 1 mm of a 20 mm diameter ball bearing to be hardened.

Answer 1

required time is 5.7 seconds

Step-by-step explanation:

solution

we first find here biot number by given formula that is

Bi =
`(hL)/(k)`

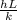
put here h is coefficient = 5000 and k = 50 W/m K and L is radius =
`(0.02)/(2)` = 0.01

so

Bi =
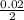`(5000 * 0.01)/(50)`

Bi = 1

so for obtaining coefficient by table from term approx for the sphere at biot no 1

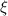`\xi` = 1.5708 and

C = 1.2732

so

and

center line temp. is here

θ* =
`(\theta)/(\theta1)`

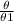
θ* =
`(T - Tx)/(T1 - Tx)`

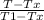
here T is 1000 K and Tx = 1300 K and T1 = 300 K

so

θ* =
`(1000 - 1300 )/(300-1300)`

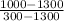
θ* = 0.3

so

radius for steel ball bearing ratio is here

r* =
`(r)/(ro)`

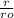
here r is 10 -1 and ro is 10

so

r* =
`(10-1)/(10)`

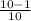
r* = 0.9

so that

fourier no for term approx for sphere is here

Fo =
`(-1)/(\xi^2) ln[ (\theta*)/(C) (1)/(\xi * r) sin ( \xi * r)]` ......1

put here value

Fo =
`(-1)/(1.5708^2) ln[ (0.3)/(1.2732) (1)/(1.5708*0.9) sin (1.5708*0.9 *(180)/(\pi))]`

Fo = - 0.4053 ln ( 0.16462)

Fo = 0.7312

so time fpr 1 mm to 20 mm dia

Fo =
`(\alpha*t)/(r^2)`

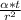
Fo =
`(kt)/(\rho C r^2)`

0.7312 =

t = 5.703 sec

so required time is 5.7 seconds