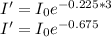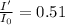We know that,

With the dates that we have, we can calculate \mu for the bone and for the tissue, that is


And for the bone,


So, calculate I for left side,
For the tissue part,
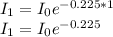
FOr the bone part,

And,

Final Intensity for the left side is given by,
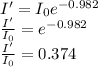
Moreover for the right side