Question

a moatboat leavs a horabor and travels at an average speed of 7 mph towards a small islamd. Six houra later, a cabain crusier leaves the same habor at an average speed of 21 mph towar the same island. In how many hors afterr the cabina crusiser leaves the harbor will it be alongside the momtorboat?

Answer 1

After 3 hours.

Step-by-step explanation:

This is a encounter problem.

We start by writing the position equation of both objects.

We assume that acceleration is equal to zero in both boats ⇒ They move with constant speed

We put the origin of coordinates in the harbour so after 6 hours the first boat travels
`7(mi)/(h).6h=42mi`

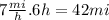
The first boat will be 42 mi far from the harbour.

The position equation for a motion with acceleration equal to zero is :

`x(t)=x_(0)+v(t-t_(i))`

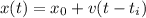
Where
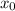`x_(0)` is initial position

v is the speed

t is time and
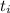`t_(i)` is initial time

Then
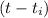`(t-t_(i))` is time variation

For the first boat :

`x1(t)=42mi+7(mi)/(h).t`

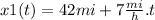
Because we set
`t_(i)=0 h`

For the second one :

`x2(t)=21(mi)/(h).t`

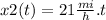
In the encounter : x1(t) = x2(t) ⇒

`42mi+7(mi)/(h).t=21(mi)/(h).t`

`14(mi)/(h).t=42mi\\ t=(42)/(14).h\\ t=3h`

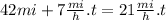
So after 3 hours they have the same position relative to the harbour

We can check by replacing the value t = 3h in both position equations

For x1(t) :

`x1(3h)=42mi+7(mi)/(h).(3h) \\x1(3h)=63mi`

For x2(t) :

`x2(3h)=21(mi)/(h).(3h)\\x2(3h)=63mi`