Answer:
Joe's Plumbing charges more per hour ($45) than Mark's Plumbing (S40)
Explanation:
Let
t ------> the number of hours
J(t) ---->the total amount in dollars that Joe's Plumbing charges
M(t) ---->the total amount in dollars that Mark's Plumbing charges
we know that
The linear equation in slope form is equal to

where
m is the slope or rate of the linear equation
b is the y-intercept (initial value)
In this problem we have
Joe's Plumbing
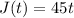
The slope or rate is equal to
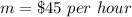

Mark's Plumbing
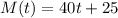
The slope or rate is equal to
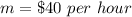
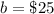
therefore
Joe's Plumbing charges more per hour ($45) than Mark's Plumbing (S40)