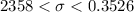Question

Use the given information to find the number of degrees of​ freedom, the critical values chi Subscript Upper L Superscript 2 and chi Subscript Upper R Superscript 2​, and the confidence interval estimate of sigma. It is reasonable to assume that a simple random sample has been selected from a population with a normal distribution. Nicotine in menthol cigarettes 80​% ​confidence; nequals22​, sequals0.28 mg.

Answer 1

Answer: Degree of freedom =
`df=21`

Critical values :

`\chi^2 _(\alpha/2 , df)=29.6151`

`\chi^2 _(1-\alpha/2 , df)=13.2396`

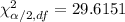
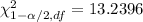
Confidence interval :
`2358<\sigma<0.3526`

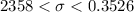
Explanation:

We know that the confidence interval for population standard deviation
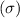`(\sigma)` is given by :-

`\sqrt{((n-1)s^2)/(\chi^2_(\alpha/2))}<\sigma<\sqrt{((n-1)s^2)/(\chi^2_(1-\alpha/2))}`

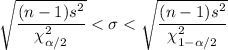
, where n = Sample size.

s= sample standard deviation.

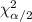`\chi^2_(\alpha/2)` and
`\chi^2_(1-\alpha/2)` = Critical values.

Given : Confidence level : c= 80%=0.80

Then, significance level =
`\alpha=1-0.80=0.20`

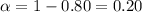
Sample size : n= 22

Degree of freedom =
`df=n-1=22-1=21`

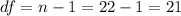
Then, by using Chi-square distribution table ,

`\chi^2 _(\alpha/2 , df)=\chi^2_{{0.10,\ 21}=29.6151`

`\chi^2 _(1-\alpha/2 , df)=\chi^2_{{0.90,\ 21}=13.2396`

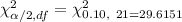
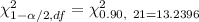
Sample standard deviation is given to be s= 0.28 mg.

Then , the 80% confidence interval estimate of
`\sigma` will be :-

`\sqrt{((21)(0.28)^2)/(29.6151)}<\sigma<\sqrt{((21)(0.28)^2)/(13.2396)}\\\\=√(0.05559326)<\sigma<√(0.12435421)\\\\=0.23578223<\sigma<0.3526389\\\\\approx0.2358<\sigma<0.3526`

Hence, the required confidence interval :
`2358<\sigma<0.3526`

Answer 2

0.16 <\sigma <0.13

Explanation:

given

S=0.28

n=22

d_f=n-1 =22-1 =21

`\chi_(L)^(2)=\chi _(0.995)^(2)`=64.278

`\chi_(U)^(2)=\chi _(0.005)^(2)`=96.578

now

80% confidence interval for Standard deviation is given by

`\sqrt{((n-1)S^(2))/(\chi _(U)^(2))}<\sigma<\sqrt{((n-1)S^(2))/(\chi _(L)^(2))}`

=
`\sqrt{((22-1)(0.28)^(2))/(64.27)}<\sigma<\sqrt{((22-1)(0.28)^(2))/(96.578)}`

=0.16 <\sigma <0.13