Question

On a certain hot​ summer's day, 677 people used the public swimming pool. The daily prices are $ 1.50 for children and $ 2.00 for adults. The receipts for admission totaled $ 1188.00 .  How many children and how many adults swam at the public pool that​ day?

Answer 1

The number of Adult swam = 345

The number of children swam = 332

Explanation:

Given in question as ,

Total number of people = 677

Total amount for admission = $ 1188.00

The daily price for children = $1.50

The daily price for adult = $2.00

Let the number of Adult = A

The number of children = C

Now ,

The number of Adult + The number of children = 677

Or, A + C = 677

And 2 A + 1.50 C = 1180

Solve both the equation , 2 A + 2 C = 1354

2 A + 1.50 C = 1188

Or , (2 A + 2 C) - (2 A + 1.50 C ) = 3154 - 1188

Or, 0.5 C = 166

Or, C =
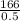`(166)/(0.5)` = 332

Total number of Children swam = 332

Again put this value in above eq,

So , A + 332 = 677

Or, A = 677 - 332 = 345

Total number of Adult swam = 345

Hence , Total number of Children swam = 332

And Total number of Adult swam = 345