Question

Find an equation of the line is the perpendicular to 30X-5Y=5 and passes through the point (0,-3).

Answer 1

it is C

Explanation:

i did the test got 100

Answer 2

`y = ((-1)/(6))x - 3`

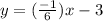
Explanation:

Given line = 30x - 5y = 5 or y = 6x + 1

so, the slope of the given line is 6.

now, let the line which is perpendicular to the given line be y = mx + c

where,

m = slope of the line

c = constant

As we know, if two lines are perpendicular to each other, the value of product of there slopes are -1.

so, slope of given line × slope of perpendicular line = -1

⇒ 6(m) = -1

⇒ m =
`(-1)/(6)`

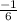
By substitutiong the value of m in the equation, we get;

⇒
`y = ((-1)/(6))x + c`

For c,

as the point (0,-3) passes through the line, we get;

⇒
`-3 = ((-1)/(6))(0) + c`

⇒
`c = -3`

Hence,

The line which is perpendicular to the given line and passes through (0,-3) is
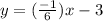`y = ((-1)/(6))x - 3` .