Question

Brenna cycles 8 kilometers during each trip to work. Write an equation that shows the relationship between the number of trips to work x and the total distance cycled y.

Answer 1

`y=8x`

Explanation:

Given:

Distance cycled by Brenna for 1 trip =
`8 \textrm{ km}`

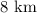
Total number of trips =
`x`

Total distance cycled for
`x` trips =
`y`

∵ Distance cycled for 1 trip =
`8 \textrm{ km}`

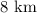
∴ Distance cycled for
`x` trips =
`8* x=8x`

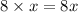
But, distance cycled for
`x` trips =
`y`.

So,
`y = 8x`.

Therefore, the relationship between the number of trips to work
`x` and the total distance cycled
`y` is
`y=8x`.