Question

A physical fitness association is including the mile run in its secondary-school fitness test. The time for this event for boys in secondary school is known to possess a normal distribution with a mean of 440 seconds and a standard deviation of 50 seconds. Find the probability that a randomly selected boy in secondary school can run the mile in less than 325 seconds. A) .4893 B) .0107 C) .9893 D) .5107

Answer 1

Answer: B) 0.0107

Explanation:

As per given , we have

`\mu=440\ \text{seconds}\\\\\sigma=50\text{ seconds}`

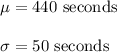
Let x be the random variable that represents the time for this event for boys in secondary school .

z-score =
`z=(x-\mu)/(\sigma)`

z-score for x= 325 ,
`z=(325-440)/(50)=-2.3`

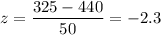
Required probability :
`P(z<-2.3)=1-P(z<2.3)=1-0.9892759\\\\=0.0107241\approx0.0107`

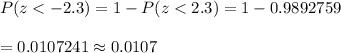
Hence, the probability that a randomly selected boy in secondary school can run the mile in less than 325 seconds = 0.0107