Question

A rocket test sled accelerates at a constant rate of 25 m/s2 from rest while moving along a straight track. The rocket engine turns off instantly when the sled reaches a speed 37 m/s. The sled then moves freely (acceleration = 0) for a period of 7 s, after which the brakes are automatically applied giving a constant deceleration of -15 m/s2. What is the total distance that the sled moves?

Answer 1

332.01 m

Step-by-step explanation:

The total distance be the
`s = s_1+s_2+s_3`

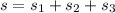
Case : 1 :- Acceleration

u = 0 m/s

v = 37 m/s

a = 25 m/s²

So, Applying equation of motion as:

`v^2-u^2=2as\\\Rightarrow s=(v^2-u^2)/(2a)\\\Rightarrow s=(37^2-0^2)/(2* 25)\\\Rightarrow s_1=27.38m`

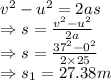
Case : 2 :- No Acceleration

t = 7 s

v = 37 m/s

`s_2=v* t\\\Rightarrow s=37* 7\ m\\\Rightarrow s=259\ m`

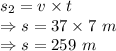
Case : 3 :- Deceleration

a = -15 m/s²

u = 37 m/s

v = 0 m/s

So, Applying equation of motion as:

`v^2-u^2=2as\\\Rightarrow s=(v^2-u^2)/(2a)\\\Rightarrow s=(0^2-37^2)/(2* -15)\\\Rightarrow s=45.63\ m`

Hence, S = 27.38 + 259 + 45.63 m = 332.01 m