Answer:
The mean nicotine content with a 95% confidence interval is (25.52mg, 26.88mg).
Explanation:
The sample size is 95.
The first step to solve this problem is finding our degrees of freedom, that is, the sample size subtracted by 1. So
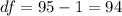
Then, we need to subtract one by the confidence level
 and divide by 2. So:
and divide by 2. So:
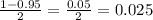
Now, we need our answers from both steps above to find a value T in the t-distribution table. So, with 94 and 0.025 in the t-distribution table, we have
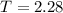 .
.
Now, we find the standard deviation of the sample. This is the division of the standard deviation by the square root of the sample size. So
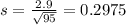
Now, we multiply T and s
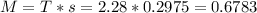
For the lower end of the interval, we subtract the mean by M. So 26.2 - 0.6783 = 25.52mg
For the upper end of the interval, we add the mean to M. So 26.2 + 0.6783 = 26.88mg
The mean nicotine content with a 95% confidence interval is (25.52mg, 26.88mg).