Question

The U.S. Bureau of Labor Statistics reports that of persons who usually work full-time, the average number of hours worked per week is 43.4. Assume that the number of hours worked per week for those who usually work full-time is normally distributed. Suppose 12% of these workers work more than 48 hours. Based on this percentage, what is the standard deviation of number of hours worked per week for these workers?

Answer 1

The standard deviation of number of hours worked per week for these workers is 3.91.

Explanation:

Problems of normally distributed samples can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by

`Z = (X - \mu)/(\sigma)`

After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X. Subtracting 1 by the pvalue, we This p-value is the probability that the value of the measure is greater than X.

In this problem we have that:

The average number of hours worked per week is 43.4, so
`\mu = 43.4`.

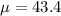Suppose 12% of these workers work more than 48 hours. Based on this percentage, what is the standard deviation of number of hours worked per week for these workers.

This means that the Z score of
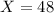`X = 48` has a pvalue of 0.88. This is Z between 1.17 and 1.18. So we use
`Z = 1.175`.

`Z = (X - \mu)/(\sigma)`

`1.175 = (48 - 43.4)/(\sigma)`

`1.175\sigma = 4.6`

`\sigma = (4.6)/(1.175)`

`\sigma = 3.91`

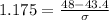
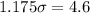
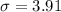
The standard deviation of number of hours worked per week for these workers is 3.91.