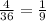Answer:
a)

b)
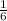
c)
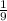
Explanation:
Total possible outcomes when a dice is rolled twice
(1,1) , (1,2) , (1,3) , (1,4) , (1,5) , (1,6)
(2,1) , (2,2) , (2,3) , (2,4) , (2,5) , (2,6)
(3,1) , (3,2) , (3,3) , (3,4) , (3,5) , (3,6)
(4,1) , (4,2) , (4,3) , (4,4) , (4,5) , (4,6)
(5,1) , (5,2) , (5,3) , (5,4) , (5,5) , (5,6)
(6,1) , (6,2) , (6,3) , (6,4) , (6,5) , (6,6)
here total number of possible outcomes is 36
now,
P (Event ) =
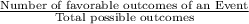
a) P( die shows a different number on each toss )
Favorable outcomes = (1,2) , (1,3) , (1,4) , (1,5) , (1,6) , (2,1) , (2,3) , (2,4) , (2,5) , (2,6), (3,1) , (3,2) , (3,4) , (3,5) , (3,6) , (4,1) , (4,2) , (4,3) , (4,5) , (4,6) , (5,1) , (5,2) , (5,3) , (5,4) , (5,6) , (6,1) , (6,2) , (6,3) , (6,4) , (6,5)
number of Favorable outcomes = 30
thus,
P( die shows a different number on each toss ) =

b) P( The sum of the dots showing is greater than or equal to 10 )
Favorable outcomes = (4,6) , (5,5) , (5,6) , (6,4) , (6,5) , (6,6)
number of Favorable outcomes = 6
P( The sum of the dots showing is greater than or equal to 10 )
=
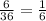
c) P( total score is less than 4 )
Favorable outcomes = (1,1) , (1,2) , (1,3) , (2,1)
number of Favorable outcomes = 4
P( total score is less than 4 ) =