Question

In incline plane makes an angle of 40° with the horizontal a body weighing 4000 N initial at rest moves down the slope calculate the distance travelled by the body in 5 seconds if the force of friction is 1500N

Answer 1

45.375m

Step-by-step explanation:

To get the distance travelled by the body we will use the equation of motion

S = ut + 1/2at²

u is the initial speed = 0m/s

a is the acceleration

t is the time = 5 seconds

We need to get the acceleration first:

According to Newton's second law,

`\sum F_x = ma\\F_m - F_f = ma\\`

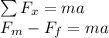
Fm is the moving force = Wsin theta

Ff is the frictional force = 1500N

m is the mass = W/g

m = 4000/9.8

m = 408.16kg

Substitute the given values in the formula and get the acceleration:

`Wsin \theta - F_f = ma\\4000sin40^0 - 1500 = 408.16a\\2,980.45 - 1500 = 408.16a\\1,480.45 = 408.16a\\a = (1,480.45)/(408.16) \\a = 3.63m/s^2`

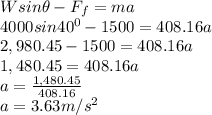
Get the required distance:

Recall that S = ut+1/2at²

S = 0(5) + 1/2*3.63*5²

S = 0+0.5*3.63*25

S = 45.375m

Hence the distance travelled by the body in 5 seconds if the force of friction is 1500N is 45.375m