Question

Calculate the amount of PV work done on the surroundings when 1.00 kg of H2O absorbs energy from sunlight and vaporizes at 1.0 atm and 25 degrees C. Assume the volume of liquid H2O is negligible (~0 m^3) compared to that of vapor. (R = 8.314 J / K-mol)

Answer 1

137639.472 J

Step-by-step explanation:

Given, Mass of water = 1 kg = 1000 g

Molar mass of water = 18.0153 g/mol

The formula for the calculation of moles is shown below:

`moles = (Mass\ taken)/(Molar\ mass)`

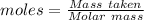
Thus,

`Moles= (1000\ g)/(18.0153\ g/mol)`

`Moles\ of\ water= 55.508\ mol`

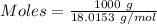
Pressure = 1.0 atm

Temperature = 25 °C

The conversion of T( °C) to T(K) is shown below:

T(K) = T( °C) + 273.15

So,

T₁ = (25 + 273.15) K = 298.15 K

Using ideal gas equation as:

`PV=nRT`

where,

P is the pressure

V is the volume

n is the number of moles

T is the temperature

R is Gas constant having value = 0.0821 L.atm/K.mol

Applying the equation as:

1.0 atm × V = 55.508 mol × 0.0821 L.atm/K.mol × 298.15 K

⇒V = 1358.7312 L

The expression for the calculation of work done by the surroundings is shown below as:

`w=P* \Delta V`

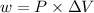
Where, P is the pressure

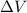`\Delta V` is the change in volume

From the question,

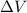`\Delta V` = 1358.7312 - 0 L = 1358.7312 L

P = 1.0 atm

`w=1.0*1358.7312\ atmL`

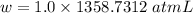
Also, 1 atmL = 101.3 J

So,

`w=1.0*1358.7312* 101.3\ J=137639.472\ J`