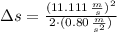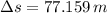Answer:
a)
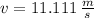 , b) See attachment below, c)
, b) See attachment below, c)
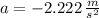 . It means that speed is decreasing at an average rate of 2.222 m/s², d) See attachment below, e)
. It means that speed is decreasing at an average rate of 2.222 m/s², d) See attachment below, e)
 , f)
, f)
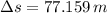 .
.
Step-by-step explanation:
a) The constant speed of the truck is:
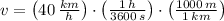
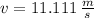
b) A simple graph is presented as attachment.
c) The average acceleration for the truck is:
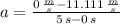
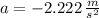
It means that speed is decreasing at an average rate of 2.222 m/s².
d) The new graph is present as attachment.
e) The time needed to regain the original speed is:


f) The distance travelled by the truck is: