Question

Concert ticket prices are $18 for a screening with 500 attendees. For each $1 increase in ticket price, attendance decreased by 5 people. What should the ticket price be to maximize revenue?

Answer 1

$59

Explanation:

Let the revenue will be maximum when $ x is increased in the ticket price.

So, the final ticket price is $(18 + x) and attendance in the concert will be (500 - 5x)

So, revenue R = (18 + x) (500 - 5x) = 9000 + 410x - 5x².......... (1)

Condition for R to be maximum is
`(dR)/(dx)  =0`

So, differentiating equation (1) with respect to x on both sides

`(dR)/(dx) =410-10x = 0`

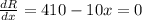
⇒ x = 41

So, the ticket price for maximum revenue is $(18 + 41) = $59 (Answer)