Answer:
He will have 175 stamps after 15 weeks
Explanation:
After 3 weeks he has collected 35 different stamps
(3,35)
After 9 weeks he has collected 105 different stamps
(9,105)
We are given that supposed to find how many stamps will he have after 15 weeks
Formula :
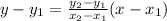

Substitute the values in the formula :
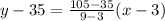

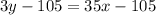
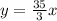
y is the no. of stamps
x is the no. of weeks
Substitute x =15
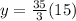
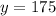
Hence he will have 175 stamps after 15 weeks