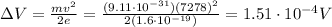Question

Please can someone help me with this question.

Your help will be much appreciated.

Q: Through what potential difference must electrons be accelerated so that they will exhibit wave nature in passing through a pinhole 0.1 in diameter

Answer 1

`1.51\cdot 10^(-4)V`

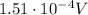
Step-by-step explanation:

The de Broglie wavelength of the electron is given by

`\lambda=(h)/(mv)`

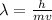
where

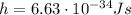`h=6.63\cdot 10^(-34) Js` is the Planck constant

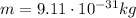`m=9.11\cdot 10^(-31) kg` is the mass of the electron

v is its speed

We want the electron to have a wavelength of

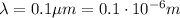`\lambda = 0.1 \mu m = 0.1\cdot 10^(-6)m` (the diameter of the pinhole)

Substituting in the equation above, we find what speed the electron should have:

`v=(h)/(m\lambda)=(6.63\cdot 10^(-34))/((9.11\cdot 10^(-31))(0.1\cdot 10^(-6)))=7278 m/s`

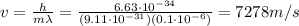
Now, when a charged particle is accelerated through a potential difference, the kinetic energy it gains is equal to the change in electric potential energy, therefore:

`e\Delta V = (1)/(2)mv^2`

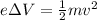
where

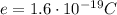`e=1.6\cdot 10^(-19) C` is the electron charge

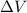`\Delta V` is the potential difference

And solving for
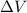`\Delta V`,

`\Delta V=(mv^2)/(2e)=((9.11\cdot 10^(-31))(7278)^2)/(2(1.6\cdot 10^(-19)))=1.51\cdot 10^(-4)V`