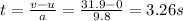Answer:
Its final velocity and how much time it takes to reach the water
Step-by-step explanation:
The motion of the stone is a uniformly accelerated motion, so we can use the following suvat equation to determine its final velocity:
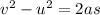
where
v is the final velocity
u = 0 is the initial velocity
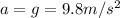 is the acceleration of gravity
is the acceleration of gravity
s = 52 m is the distance covered during the fall
Solving for v,
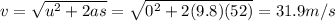
We can also find how much time it takes to reach the water, using the equation

where
v = 31.9 m/s is the final velocity
u = 0 is the initial velocity
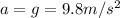
t is the time
And solving for t,