Question

Phoebe threw a frisbee horizontally that traveled 125 m. the frisbee left her hand traveling at 45 m/s. as the frisbee travels in the air it slows down with a deceleration of 5.6 m/s2. How long was the frisbee in the air? When Mike caught it, how fast was it traveling?

Answer 1

1) 3.57 s

The motion of the frisbee is a uniformly accelerated motion, so we can use the suvat equation:

`s=ut+(1)/(2)at^2`

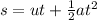
where

s is the displacement

u is the intiial velocity

t is the time

a is the acceleration

Here we have

s = 125 m (distance travelled)

u = 45 m/s (initial velocity)

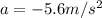`a=-5.6 m/s^2` (deceleration of the frisbee)

Substituting into the equation, we get

`125 = 45t-2.8t^2\\2.8t^2-45t+125=0`

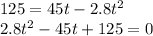
The solution of this equation are:

`t=(-b\pm √(b^2-4ac))/(2a)=(-(-45) \pm √((-45)^2-4(2.8)(125)))/(2(2.8))`

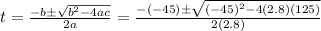
Which gives two solutions:

t = 3.57 s

t = 12.50 s

So, the frisbee crosses the point at distance d = 125 m from Phoebe two times: at 3.57 s the first time, then if it is free to move, the frisbee continues its motion and then at some point moves back to this point at t = 12.5 s. Since we are interested in the time at which Mike caught the frisbee, we just take the first solution:

t = 3.57 s

2) 25 m/s

Since this is a uniformly accelerated motion, the velocity of the frisbee is given by

`v=u+at`

where we have

u = 45 m/s is the initial velocity

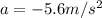`a=-5.6 m/s^2` is the acceleration of the frisbee

Since the frisbee has been caught at time

t = 3.57 s

We can substitute this value into the equation to find the final velocity of the frisbee:

`v=45+(-5.6)(3.57)=25.0 m/s`

Answer 2

The frisbee was in air for 12.5 seconds and when mike caught it it's speed was = 10m/s

Explanation:

we know that distance travelled horizontally is given by

`\mathrm{s}=\mathrm{u} \mathrm{t}+1 / 2 * \mathrm{a} t^(2)`

where s = total distance travelled = 125 (given),

u = initial velocity = 45m/s (given) ,

a = acceleration = -5.6
`m/s^2` (since we are given deceleration a = negative) and

t = total travel time

substituting these values in the formula

125 = 45*t + (1/2)(-5.6)
`t^2`

we get t = 12.5 and 3.578... since 12.5 is more accurate value

we take t = 12.5 which gives the time for which frisbee stays in air

also we know speed =
`\frac{\text { distance }}{\text { time }}`

since we are given total distance = 125m

and total time we have found, t = 12.5

we get speed =
`(125)/(12.5)=10`

= 10m/s is how fast the frisbee was when mike caught it.