Question

Austin is arranging 14 cans of food in a row on a shelf. He has 5 cans of corn, 1 can of olives, and 8 cans of beans. In how many distinct orders can the cans be arranged if two cans of the same food are considered identical (not distinct)?

Answer 1

18018

Explanation:

Given:

Number of cans = 14

Number of cans of corn = 5

Number of cans of olives = 1

Number of cans of beans = 8

When there are
`n` objects to be arranged in order, out of which
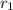`r_(1)` objects are of one kind,
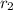`r_(2)` objects are of another and so on, then the number of different arrangements is given as:

`(n!)/(r_(1)!* r_(2)!* ...r_(k)!)`

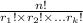
Here,
`n = 14, r_(1)=5,r_(2)=8`

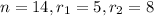
Therefore, the distinct orders in which the cans can be arranged is
`(14!)/(5! * 8!) = 18018`