Answer: The final velocity of goalie and ice puck is 0.075 m/s in opposite direction
Step-by-step explanation:
To calculate the velocity of the goalie and ice puck after the collision, we use the equation of law of conservation of momentum, which is:
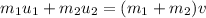
where,
 = mass of goalie = 70.0 kg
= mass of goalie = 70.0 kg
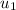 = Initial velocity of goalie = 0 m/s
= Initial velocity of goalie = 0 m/s
 = mass of ice puck = 0.150 kg
= mass of ice puck = 0.150 kg
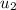 = Initial velocity of ice puck = 35.0 m/s
= Initial velocity of ice puck = 35.0 m/s
 = Final velocity of goalie and ice puck = ?
= Final velocity of goalie and ice puck = ?
Putting values in above equation, we get:
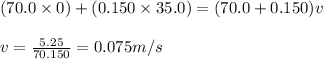
Hence, the velocity of goalie and ice puck is 0.075 m/s in opposite direction