Question

A series circuit has a capacitor of 0.25 × 10−6 F, a resistor of 5 × 103 Ω, and an inductor of 1 H. The initial charge on the capacitor is zero. If a 24-volt battery is connected to the circuit and the circuit is closed at t = 0, determine the charge on the capacitor at any time t.

Answer 1

`q_c(t) = C_1e^((-4* 10^3)t)+C_2e^((- 10^3)t)`

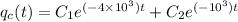
Explanation:

given,

C = 0.25 × 10⁻⁶ F

R = 5 × 10³ F

L = 1 H

E = 24

the equation of the circuit

`L(d^2q)/(dt^2)+R(dq)/(dt)+(q)/(C)=E`

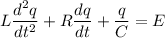
substitute the given data

`(1)(d^2q)/(dt^2)+(5 * 10^3)(dq)/(dt)+(q)/(0.25* 10^6)=24`

`(d^2q)/(dt^2)+(5 * 10^3)(dq)/(dt)+(4* 10^6)q=24`

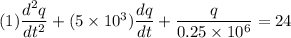
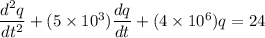
auxiliary equation

`m^2+(5 * 10^3)+(4* 10^6) = 0`

`m = (-5* 10^3 \pm √((5* 10^3)^2-4* 4* 10^6))/(2)`

`m = (-5* 10^3 \pm √(25* 10^6-16 10^6))/(2)`

`m = -4 * 10^3,-10^3`

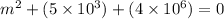
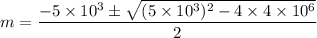
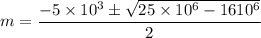
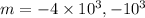
so, complimentary function

`q_c(t) = C_1e^((-4* 10^3)t)+C_2e^((- 10^3)t)`

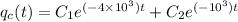
charge at any time t is given by the above equation.

C₁ and C₂ are arbitrary constant

which will be calculated using boundary condition.