Answer:
Given system of linear equation 4y – 36 = -12x and 3x + y = -9 do not have any solution.
Solution:
Need to determine solution of following system of linear equations
4y – 36 = -12x
3x + y = -9
lets modify given equation in standard form
12x + 4y -36 = 0 -------(1)
3x + y + 9 = 0 -------(2)
Lets first analyze whether given system of equation is having solution or not.
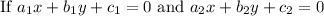 are two equation and
are two equation and
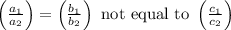 then the given system of equation has no solution
then the given system of equation has no solution
In our case
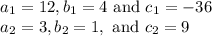
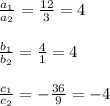
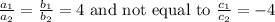
This is condition of no solution.
Hence given system of linear equation 4y – 36 = -12x and 3x + y = -9 do not have any solution.