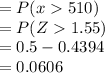Answer:
Explanation:
Let X be number of passengers will be on a commuter train
Given that X is normal with mean =476 and std deviation=22
Or we can say that
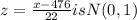
a) Probability that the number of passengers will be Between 476 and 500 passengers
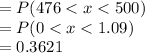
b) Probability that the number of passengers Less than 450 passengers

c) Probability that the number of passengers More than 510 passengers