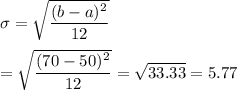Answer:
a) 0.75
b) 0.5
c) Mean = 60 minutes
Standard Deviation = 5.77 minutes
Explanation:
We are given the following information in the question:
The cycle time for trucks hauling concrete to a highway construction site is uniformly distributed over the interval 50 to 70 minutes.
a = 50, b = 70
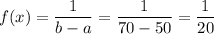
We are given a uniform distribution.
a) P(cycle time will be less than 65 minutes)
P( x < 65)
![=\displaystyle\int_(65)^(50) f(x) dx\\\\=\displaystyle\int_(65)^(50) (1)/(20)dx\\\\=(1)/(20)[x]_(65)^(50) = (1)/(20)(65-50) = 0.75](https://img.qammunity.org/2020/formulas/mathematics/high-school/ef7x35b6jah8uj5ny4nmtzgrizcv613wbb.png)
b) P(cycle time is less than 65 minutes if it is known that the cycle time exceeds 55 minutes)
P( 55 < x < 65)
![=\displaystyle\int_(65)^(55) f(x) dx\\\\=\displaystyle\int_(65)^(55) (1)/(20)dx\\\\=(1)/(20)[x]_(65)^(55) = (1)/(20)(65-55) = 0.5](https://img.qammunity.org/2020/formulas/mathematics/high-school/9kmjahvt6q1qr1sv13w3ovktoplb6jx0qk.png)
c) Mean:
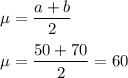
Standard Deviation: