Question

A racecar driver is driving her car down the drag strip at 120 m/s. What is the shortest distance in which she can brake and stop if the coefficient of static friction between the tires and the road is 0.71?

Answer 1

1034.5 m

Step-by-step explanation:

To calculate the displacement of the car down the drag strip, we have to find its acceleration, which is affected by gravity and the coefficient of static friction. So, we calculate the acceleration first:

`a=-\mu g = -(0.71)(9.8(m)/(s^(2) ) )=-6.96(m)/(s^(2) )`.

As you can see, the friction, virtually, makes gravity decrease, that is, the acceleration on the car is less.

Then, we use cinematic equations to find the displacement:

`v_(final)^(2)  =v_(inicial)^(2) +2ad`

Isolating d and replacing all values:

`d=(v_(final)^(2)-v_(inicial)^(2))/(2a) = (0 - (120m/s)^(2) )/(2(-6.96(m)/(s^(2) ))) \\d= 1034.5 m`

Therefore, the shortest distance is d= 1034.5 m.

Answer 2

1034.78 m

Step-by-step explanation:

The shortest distance is the displacement of the car from initial position to final position.

Displacement of body is given using Newton's equations of motion.

Given:

Initial velocity,
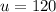`u = 120` m/s

Final velocity,
`v = 0` m/s( As the car stops in its final position)

Coefficient of static friction,
`\mu=0.71`

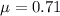
Acceleration due to gravity,
`g=9.8\textrm{ } m/s^(2)`

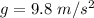
Now, when brakes are applied, only friction acts on the body in a direction opposite to that of its motion.

The acceleration of the car when friction acts the stopping force is given as:

`a=- \mu g`.

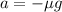The acceleration is negative as it reduces the velocity of the motion and acts in the direction opposite to that of the motion.

Plug in 0.71 for
`\mu` and 9.8 m/s² for
`g`. Solve for
`a`.

So,
`a=-0.71* 9.8=-6.958\textrm{ }m/s^(2)`.

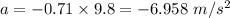Now, displacement of the car is given using the following equation of motion:

`v^(2)=u^(2) +2aS`

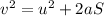
Here,
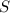`S` is the displacement of the racing car.

Plug in 120 m/s for
`u`, 0 m/s for
`v`, -6.958 m/s² for
`a`. Solve for
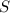`S`. This gives,

`0^(2) =(120)^(2)+2(-6.958)S\\13.916S=14400\\S=(14400)/(13.916)=1034.78\textrm{ m}`

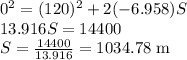
Therefore, the shortest distance in which she can brake and stop is 1034.78 m.