Question

A block of wood has a small metal disk attached to one side, small enough that the average density of the block is still less than the density of water. If you carefully place the block into water with the metal disk on top and so out of the water then it will float. If you now turn the block over so that the metal is on the bottom and so in the water will the block still float, and if so will it float at the same level, or higher, or lower? (Don’t forget to justify your answer.)

Answer 1

here no change in the level and the system will still float with same level

Step-by-step explanation:

When metal disc is on upper side of the wooden cube

then we will have

`Weight = Buoyancy`

`Mg = \rho V g`

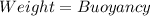
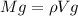
here V = submerged volume

now we have

`V = (M)/(rho)`

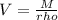
M = total mass of disc and wooden cube

`\rho` = density of water

now when we put the disc downwards then also we know that mass will remain the same

so submerged volume will be given as

`V = (M)/(\rho)`

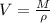
so here no change in the level and the system will still float with same level