Answer:
Part a) m∠A=72°
Part b) m∠B=108°
Explanation:
we know that
In a parallelogram opposite angles are congruent and consecutive angles are supplementary
so
In this problem
m∠A=m∠C
m∠A+m∠B=180°
step 1
Find the value of y
m∠A=m∠C
substitute the given values
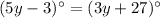
solve for y
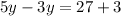
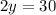
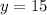
step 2
Find the measure of angle A
m∠A=(5y-3)°
substitute the value of y
m∠A=(5(15)-3)=72°
step 3
Find the measure of angle B
m∠A+m∠B=180°
we have
m∠A=72°
substitute
72°+m∠B=180°
m∠B=180°-72°
m∠B=108°