Question

Cindy has 26 nickels. She is getting rolls of nickels from the bank. She has enough money to get up to 10 rolls of nickels and each roll contains 40 nickels. The bank will not give partial rolls. The function that models the number of nickels Cindy will have after leaving the bank is f(r)=40r+26, where r is the number of rolls of nickels she gets.

What is the practical domain of the function?



a) {66, 106, 146, 186, 226, 266, 306, 346, 386, 426}
b) all integers from 1 to 10, inclusive
c) all real numbers
d) r>1

Answer 1

(b) all integers from 1 to 10, inclusive

Explanation:

While the theoretical domain encompasses all possible solutions (i.e., all real numbers), the practical domain is this case is (b) all integers from 1 to 10, inclusive. That's because the practical domain narrows the solution set to be realistic within the parameters defined by the problem. In other words, Cindy is limited to buying whole rolls of nickels only, which indicates that r must be an integer. Also, while Cindy is buying at least one roll of nickles, she is limited to buying no more than 10 rolls, which indicates that
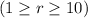`(1 \geq r \geq 10)`.

Answer 2

C) All Real Numbers

Explanation:

The domain of the expression is all real numbers except where the expression is undefined. In this case, there is no real number that makes the expression undefined.

Interval Notation: ( − ∞ , ∞ )

Set-Builder Notation: x ∈ R